Buckminster Fuller's Vector Equilibrium and the Magic of 12

Buckminster Fuller, a renowned 20th-century architect, systems theorist, and inventor, introduced the concept of the vector equilibrium as part of his synergetic geometry. This post explores the vector equilibrium, its properties, and its significance in Fuller's work, with a particular focus on the role of the number 12 in its structure.

What is the Vector Equilibrium?

The vector equilibrium, also known as the cuboctahedron, is a polyhedron with 8 triangular faces and 6 square faces. In Fuller's conceptualization, it represents a state of perfect equilibrium where all vectors are of equal length and all forces are balanced.

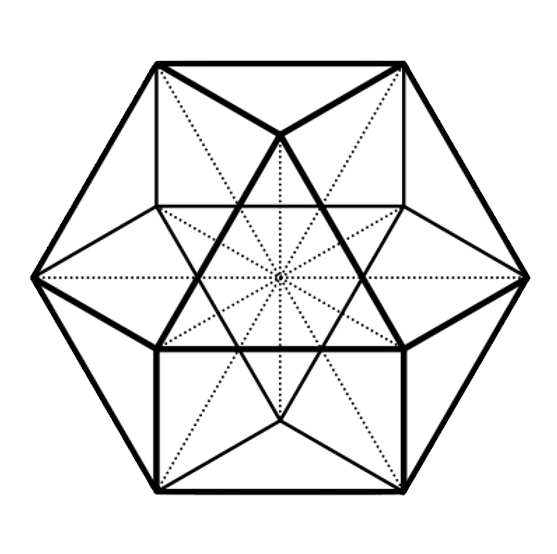
Key properties of the vector equilibrium include:

1. It has 12 identical vertices

2. It consists of 24 edges (or vectors) of equal length

3. Its 14 faces are composed of 8 equilateral triangles and 6 squares

The Significance of 12 in the Vector Equilibrium

The number 12 appears repeatedly in the structure of the vector equilibrium:

1. 12 Vertices: Each vertex connects four edges, creating a symmetrical distribution of forces.

2. 12 Axes of Symmetry: The shape has 12 axes around which it can be rotated to produce an identical configuration.

3. 12 Closest Packed Spheres: When spheres are closest-packed around a central sphere of the same size, 12 spheres fit perfectly.

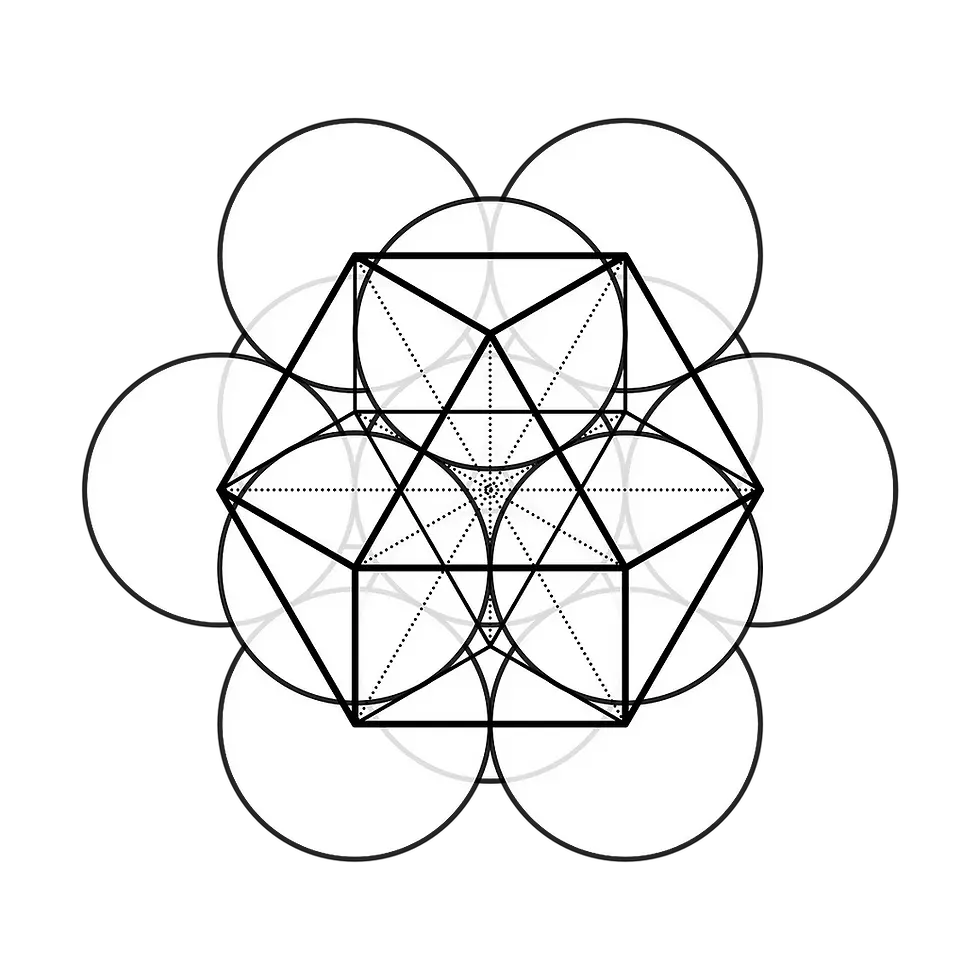
Mathematical Properties

The vector equilibrium possesses several interesting mathematical properties:

1. Volume: Its volume is 20 times the volume of a tetrahedron with edges of the same length.

2. Surface Area: The surface area is 14 times the square of the edge length.

3. Dual Polyhedron: Its dual is the rhombic dodecahedron, which also plays a significant role in Fuller's geometry.

Fuller's Interpretation and Application

Fuller saw the vector equilibrium as more than just a geometric shape. For him, it represented:

1. Energy Distribution: A model for how energy distributes itself in space.

2. Structural Integrity: A basis for understanding structural integrity in architecture and engineering.

3. Natural Systems: A reflection of patterns found in natural systems, from molecular structures to cosmic arrangements.

Practical Applications

The principles derived from the study of the vector equilibrium have found applications in various fields:

1. Architecture: Influencing the design of geodesic domes and other efficient structures.

2. Materials Science: Informing the study of molecular and crystal structures.

3. Systems Theory: Providing a model for understanding complex systems and their interactions.

Conclusion

Buckminster Fuller's vector equilibrium concept offers a unique perspective on geometry, structure, and systems. By understanding its properties, particularly the recurring significance of the number 12, we gain insight into Fuller's broader ideas about the fundamental organization of the universe. While it may not explain everything about how the world works, it provides a valuable tool for thinking about balance, symmetry, and interconnectedness in both natural and human-made systems.